Think you’ve got your head wrapped around Continuity of Functions? Put your knowledge to
the test. Good luck — the Stickman is counting on you!
Q. Which of the following statements is true?
A continuous function on a closed interval [a, b] must be bounded.
A bounded function on a closed interval [a, b] must be continuous.
A continuous function on an open interval (a, b) must be bounded.
A bounded function on an open interval (a, b) must be continuous.
Q. Let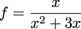 
. For which interval can we use the Boundedness Theorem to conclude that f must be bounded on that interval?
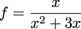. For which interval can we use the Boundedness Theorem to conclude that f must be bounded on that interval? [0, 1]
[-5, 0]
(2, 3)
[1, 2]
Q. Which of the following graphs shows a function that is both bounded and discontinuous on [a, b]?
(a) and (b)
(b) and (d)
(c) and (d)
(a), (b), and (d)
Q. A continuous function on a closed interval [a, b]
must be bounded on that interval and attain a maximum value on that interval.
must be bounded on that interval but need not attain a maximum value on that interval.
need not be bounded on that interval but must attain a maximum value on that interval.
need not be bounded on that interval and need not attain a maximum value on that interval.
Q. What is the maximum value of f(x) = sin(x) on the interval [π, 2π)?
f does not attain a maximum on this interval.
0
1
-1
Q. A continuous function on a closed interval [a, b]
must attain its maximum and minimum value exactly once each.
may attain its maximum and minimum value more than once, but only a finite number of times each.
may attain its maximum and minimum value an infinite number of times each.
need not attain a maximum or minimum value at all.
Q. Let 
. For which interval(s) can we use the Extreme Value Theorem to conclude that f must attain a maximum and minimum value on that interval?
. For which interval(s) can we use the Extreme Value Theorem to conclude that f must attain a maximum and minimum value on that interval? [0, 1]
[-1, 0]
neither [0, 1] nor [-1, 0]
both [0, 1] and [-1, 0]
Q. If f is continuous on [a, b] and f(b)< M < f(a) then the Intermediate Value Theorem tells us
how many values of c exist in (a, b) with f(c) = M.
one value of c in (a, b) at which f(c) = M.
all values of c in (a, b) at which f(c) = M.
that there is a value of c in (a, b) with f(c) = M.
Q. Let 
. On which of the following intervals does the IVT guarantee the existence of a value c with f(c) = 0?
. On which of the following intervals does the IVT guarantee the existence of a value c with f(c) = 0? (-3, -2)
(-3, -1)
(-3, 0)
(-3, 1)
Q. Which of the following pictures best illustrates the IVT?
Picture (a)
Picture (b)
Picture (c)
Picture (d)