Grade 8

Grade 8

Geometry 8.G.B.7

7. Apply the Pythagorean Theorem to determine unknown side lengths in right triangles in real-world and mathematical problems in two and three dimensions.

Once upon a long, long time ago, life was hard. There were no electronics. (Gasp!) No cell phones—not even those phones your grandparents remember with the rotary dials. There were no video games, no computers, no TV—not even radio. In fact, we're talking about a time before microwaves and even books. (Thanks for nothing, Gutenberg.)

So what did people do all day in this crazy time? Well, a bunch of the ones in Greece spent a lot of time talking about math, and they came up with a lot of the rules we now take for granted.

That's where Pythagoras comes in. This dude spent a good chunk of his time thinking about math and philosophy. He even came up with an important theorem for which he would be forever remembered: the Pythagorean Theorem. (Yes, he named it after himself. Wouldn't you if you came up with a theorem?)

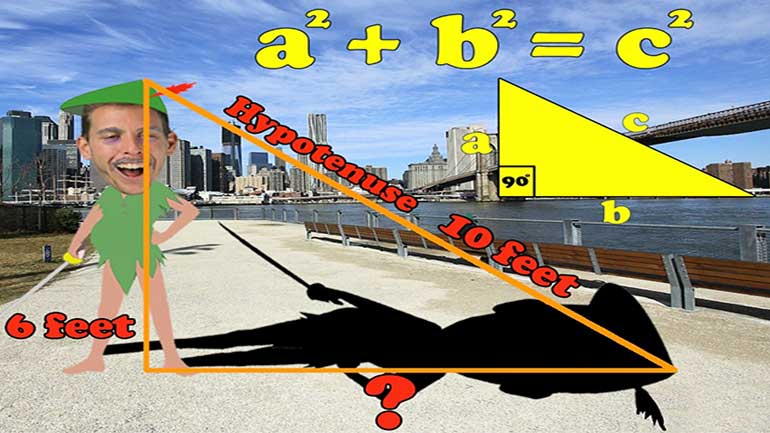
It states, basically, that if a triangle is a right triangle, then the sum of the squares of the lengths of the legs equals the square of the length of the hypotenuse. Your students probably know it by its formula: a2 + b2 = c2.

It's about time your students get up close and personal with that formula, because they're going to use it—a lot. They should know not only when to apply the formula, but how to plug in numbers. They should also be able to interpret their answers in ways that make sense. (For example, although c2 = 25 means that c = ±5, a hypotenuse of length -5 doesn't make sense!)

If your students can find missing side lengths, apply the formula to real-life scenarios, and pick out the errors that these guys make, they should be in good shape.

 

Drills

  1. What is the hypotenuse of a right triangle with legs of lengths 6 and 8?

    Correct Answer:

    10

    Answer Explanation:

    Using the Pythagorean Theorem, we can substitute 6 for a and 8 for b in the formula a2 + b2 = c2. We know to do that because we're looking for the hypotenuse, c. We find that 36 + 84 = 100 = c2. If we take the square root of 100, we get 10. (We can't have a hypotenuse of length -10, now can we?) So our answer is (B).


  2. A right triangle has a hypotenuse of length 12 and a leg of length 8. What is the length of the other leg?

    Correct Answer:

    Answer Explanation:

    In the formula a2 + b2 = c2 (because that's what this all comes down to), the hypotenuse is c and the other two legs are a and b. As long as we substitute 12 for c, it doesn't matter whether 8 is a or b. Either way, we'd get 64 + b2 = 144, or b2 = 80, which isn't a perfect square. That means (A) and (B) are out. Taking the square root and simplifying gives us (C) as our answer.


  3. Both legs of a right triangle are of length 1. What is the length of the hypotenuse?

    Correct Answer:

    Answer Explanation:

    Right off the bat, we know that (C) and (D) can't be right because a triangle of lengths 1, 1, and 2 or 1, 1, and 3 can't exist. (Ever heard of a little thing called the triangle inequality theorem?) If we use the Pythagorean Theorem, we end up with 12 + 12 = c2 = 2. Simplifying the equation would give us c =  as our answer.


  4. Which of the following is NOT a Pythagorean Triple?

    Correct Answer:

    7-15-17

    Answer Explanation:

    Unless you've memorized 'em, the only way to figure this one out is to calculate, calculate, calculate. If you use the right formula (a2 + b2 = c2) and plug the numbers in properly (the largest number is c and the others are either a or b), then you should be good to go. The only set of numbers that doesn't quite cut it is (C). All the others fit into the Pythagorean Theorem just fine. (And if they don't, try replacing that abacus with a calculator. Usually does the trick.)


  5. A rectangle has a diagonal of 50 and a base of 40. Find the height of the rectangle.

    Correct Answer:

    30

    Answer Explanation:

    Rectangles?! What do rectangles have anything to do with right triangles? Well, the diagonal of a rectangle is the hypotenuse of the right triangles that make it up. The diagonal is the hypotenuse, and the base and height are the two legs of the triangle. We can find the height by plugging in 50 for c and 40 for a. That gives us 402 + b2 = 502, and solving for b gives us 30. (It's really just a 3-4-5 triangle with dimensions multiplied by 10!)


  6. The distance between home plate and first base is 90 feet. The distance from first base to second base is also 90 feet. The angle at first base is a right angle. What's the shortest distance between home plate and second base?

    Correct Answer:

    Answer Explanation:

    If we imagine the distance from home plate to second base as the hypotenuse of a right triangle with both legs being 90 feet long, we can apply the Pythagorean Theorem to find the distance. This just means substituting a2 + b2 = c2 with 90 for both a and b. This gives us  feet, which is the shortest distance between home plate and second base. While we could go 135 feet or even 180 feet just to get from one to the other, the absolute shortest distance we could travel would be about 127 feet.


  7. After deciding to remodel his house a little, Mr. Feeney bought a 15-foot handrail for his new staircase. If the second story of the house is 12 feet above the first floor, how long (horizontally) should Mr. Feeney's staircase be?

    Correct Answer:

    9 feet

    Answer Explanation:

    We can picture Mr. Feeney's handrail as the hypotenuse of a right triangle, where the height of the staircase is one leg and its length is the other. Since we already know the length of the hypotenuse (15 feet) and the vertical leg (12 feet), we can use the Pythagorean Theorem to solve for the horizontal leg (the length of the staircase). Substituting in 12 feet for b and 15 feet for c, we can find a = 9 feet. The other answers wouldn't do the whole a2 + b2 = c2 business justice, so they wouldn't make a right triangle.


  8. Kendra wants to buy a new plasma TV for her media room. (Who doesn't?) Her entertainment center can accommodate a length of up to 50 inches and a height of 30 inches. Does she have room for a 54-inch (diagonal) model?

    Correct Answer:

    Yes, she can get the 54" TV with lots of room to spare

    Answer Explanation:

    To find out whether Kendra's TV will fit, we can compare the diagonal of the TV model to the diagonal of the entertainment center. We can do that using the Pythagorean Theorem. (Shocker.) Here, the length and height of the entertainment center (50" and 30") are the legs in our right triangle. Using our beloved a2 + b2 = c2 equation, we can find that the length of the diagonal is slightly larger than 58 inches. That leaves plenty of room for the 54" model to fit and she could even fit a 58" diagonal TV if she wanted to. Lucky Kendra.


  9. In order to support the top of a cylindrical circus tent, Bozo the Clown wants to run a support wire from the top of the tent down to the edge of the base. (He isn't good with heights, so he'll get an acrobat to do it.) The height of the tent is 30 feet and an 80-foot wire should do the trick. What is the approximate diameter of the tent?

    Correct Answer:

    148 feet

    Answer Explanation:

    If we imagine the tent cut in half, it would look like an isosceles triangle. Splitting it vertically along the center would make two identical right triangles. One of the legs would be the height of the tent (30 feet) and the other would be the distance from the middle to the edge of the tent, while the hypotenuse would be the distance from the top of the tent down to its edge—where the 80-foot wire would go. Using the Pythagorean Theorem (duh), we can find the distance from the center to the edge of the tent: about 74 feet. Don't pick (A), though! We want the diameter, which is twice that distance, making (C) our answer.


  10. Twins James and Jamie, age 5, are getting bunk beds. Their parents picked up the box from Shmikea and found that it has dimensions of 6 feet by 2 feet by 3 feet. Their minivan can only hold a box with a diagonal of 6.8 feet at most. Will the bunk bed fit in their trunk?

    Correct Answer:

    No, it won't fit in their trunk

    Answer Explanation:

    What do we do with three dimensions? We get three-dimensional—but really, we only use the Pythagorean Theorem twice.

    First, we can see that finding the hypotenuse of a right triangle with legs 6 and 3 will give us the distance across the bottom of the box. To add the height in, we'll then use that "hypotenuse" (now the leg) to find the distance across the entire box. First, 62 + 32 = 45 = c2, so our diagonal across the bottom is . Now, we can use that length and the height (2 feet) as the two legs of another right triangle: , so the diagonal is 7 feet exactly. Since it's just barely too large compared to the 6.8 feet of cargo space available in the van, (D) is wrong and (C) is the answer we want.


More standards from Grade 8 - Geometry

Tired of ads?

Join today and never see them again.
Get Started