The first thing we do, as always, is simplify each radical term if possible. The radical terms here are already simplified, so we can go straight to distributing. We distribute the first term in the first set of parentheses to the first term in the second set: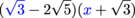 
...which gives us: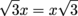 
Then distribute that first radical to the second term in the second set of parentheses: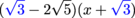 
...which gives us: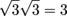 
Now we move on to distributing the second term in the first set to the first term in the second set: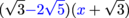 
Bam: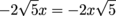 
Last but not least, we multiply the last terms: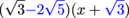 
That gives us: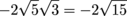 
Finally, we add the products we got from all that mad distributin':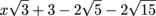 
We can't simplify this any, so we're done. Stick a fork in us. Be Careful: When multiplying radicals, write neatly so you know what's part of the radicand and what isn't. This is no time for your usual hieroglyphic-doctor-signature-chicken-scratch. In other words,  is not the same thing as  . It's easy to make that mistake, so set yourself up for success. It's safer to write  as 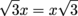or  or  or  . However you write things, make sure you understand your own notation. If you forget or can't read what you wrote, no one will be able to help you. You'll be up a radical without a paddical. | 
.
.
.
.
. Everybody inside the parentheses needs a crack at him. 
.