ShmoopTube
Where Monty Python meets your 10th grade teacher.
Search Thousands of Shmoop Videos
Pre-Algebra Videos 34 videos
ACT Math: Pre-Algebra Drill 5, Problem 2. If a and b are prime numbers, how many factors does ab have?
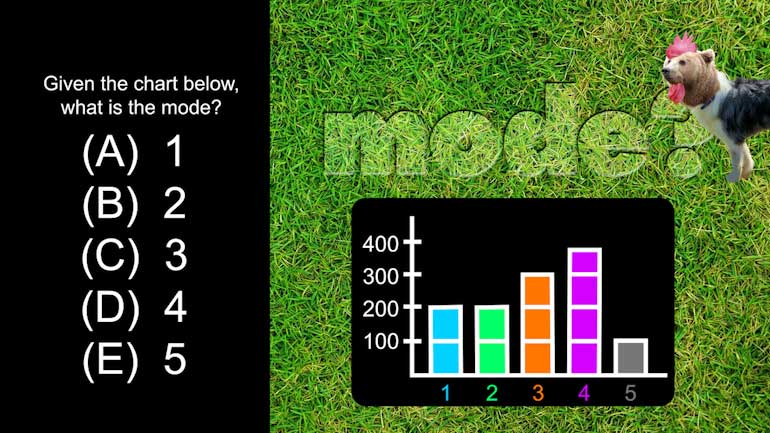
ACT Math Pre-Algebra Drill 2, Problem 5. Given the chart in the video, what is the mode?
Jenny's Overall Mean (Not Like That) 290 Views
Share It!
Description:
ACT Math: Pre-Algebra: Drill 6, Problem 2. What score must be earned to bring Jenny's overall mean to at least 87?
Transcript
- 00:03
You look like you could use a shmoopy question...
- 00:06
On her first four tests, Jenny earned an 85,
- 00:08
97, 79, and 84. Her uber-strict parents aren't letting her
- 00:13
attend the State Math Finals unless she brings her overall mean up to at least 87.
- 00:19
What must Jenny earn on her next test to be allowed to have... every young girl's dream come true?
Full Transcript
- 00:28
And here are the potential answers...
- 00:33
OK so what is this question asking?
- 00:36
Well it's really the advanced ninja version of taking averages.
- 00:40
Poor Jenny took 4 tests and she's going to take another
- 00:43
-- she will have taken 5 tests in total.
- 00:46
And the TOTAL of these 5 tests must have an average of 87 -- or in other words, they
- 00:51
must total to 5 times 87 or have a total of 435.
- 00:56
So the answer comes from just adding up the previous 4 tests, subtracting that number
- 01:02
from 435 and whatever is left is what she has to get to average an 87.
- 01:11
So if we add the previous 4 tests we get a sub-total of 345.
- 01:14
Subtract 345 from 435 and we get 90.
- 01:18
So she has to get a 90 or better on the last test.
- 01:22
Answer D.
- 01:23
As in, "Deadly weapons."
Related Videos
ACT Math: Plane Geometry Drill 3, Problem 1. What is the area of the trapezoid shape in the video?
ACT Math: Coordinate Geometry Drill 1, Problem 1. Which inequality is expressed by the number line?
ACT Math: Intermediate Algebra: Drill 3, Problem 1. Find the fifth number in the series.
Statistics, Data, and Probability I: Drill Set 3, Problem 4. How many different avatars can be created based on the given options?